Math in the Movies: A Wrinkle in Time

Geometry can be a daunting subject for many students. Imagining shapes and figures and how they fit together in the physical world can be a tough thing to grasp. But if we can make it more concrete and help students wrap their hands, as well as their eyes, around it...we can help make geometry lessons easier to understand. We can help students actually enjoy and look forward to learning!

The hit movie, A Wrinkle in Time, based on the book of the same name by Madeleine L’Engle, has kids of all ages jazzed. The story has elements of family, love, friendship, mystery, danger, and even the fight against evil. All of those are wrapped up in a plot of science, technology, and a bit of the supernatural.

Travel Through Dimensions by Wrinkling Time

The book and the movie, Wrinkle in Time, has a bit of something for everyone! No wonder it’s such a long-standing favorite. The story takes our heroes into a world of travel outside of time and space, through a tesseract. Using a tesseract, the kids in the story are able to “wrinkling time”. They can travel through space without having to go the long way around. They can jump into the fifth dimension via the tesseract and travel through dimensions, visiting planets and accomplishing their missions.

And that’s why it is a fantastic theme for this math lesson: calculating surface area and volume of 2D and 3D figures, and changing dimensions using the calculations. Geometric shapes are full of dimension. A line is one-dimensional, a plane is two-dimensional, and a solid figure is three-dimensional. The fourth dimension is an extension of three dimensional object. It’s almost like cubing a cube but it is the dimension that some say would aid in time travel. This is why a tesseract is considered to be in the fourth dimension. Thus, the fourth dimension is referred to as the transition to the fifth dimension. So you see, the idea of traveling anywhere in time and space is based in math!

The Lesson Plan

https://www.youtube.com/watch?v=2Au6HRHo1vY

First, as a class we reviewed formulas for figuring perimeter, circumference, and area for 2-dimensional shapes. Then we reviewed the surface area and volume formulas 3-dimensional shapes. For example, I demonstrated how a 2-dimensional net consisting of 6 squares can be turned into a 3-dimensional cube, then we found the surface area and volume of the cube as a class.

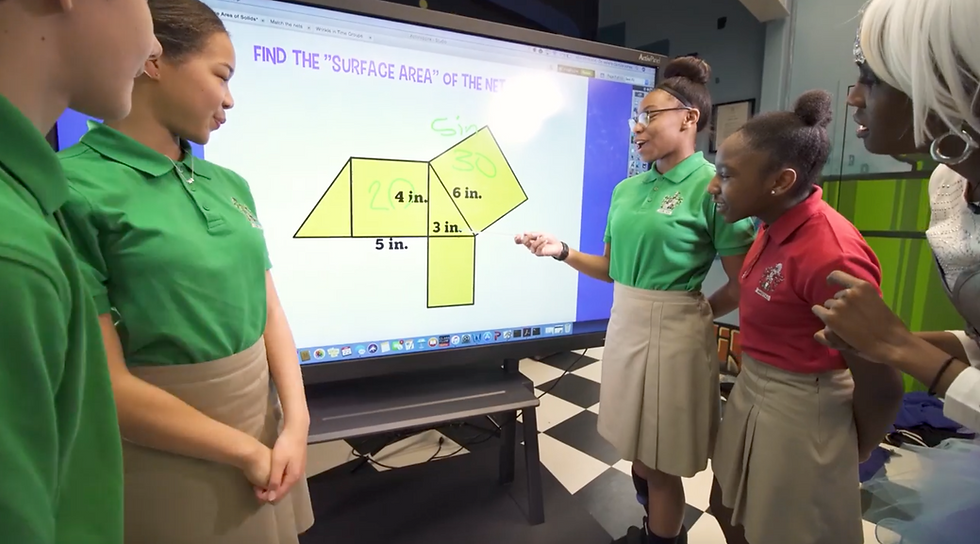
To determine how much information the students retained, we worked through a group of activities. In the first activity, the students played a matching game electronically comparing 3D figures with their 2D nets. Next, the students worked in groups to hand draw the 3D shapes based on their 2D nets. Lastly, the students worked on problems determining the perimeter and area of 2D nets and then traveled to the third-dimension find the surface area and volume of 3D shapes.

After working through the calculations, the students got the chance to build their very own tesseract: the four-dimensional analogue of the cube. My students and I loved this part of the lesson most of all. They got to work as a team to conceptually visualize a tesseract and how to construct it. Team building is also one of the many themes from A Wrinkle in Time. To conclude the activity, the students found the surface area and volume of their own life-size tesseracts.

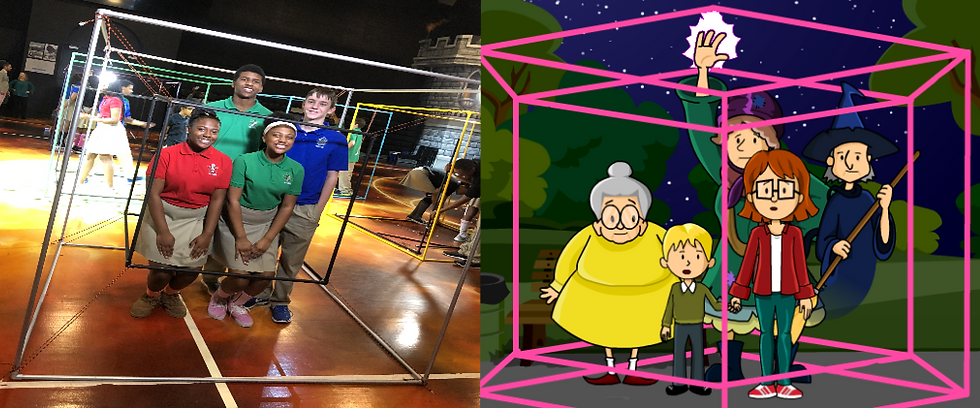
Here is the lesson plan for this activity. I hope you and your students have as much fun as we did learning about these shapes and building our tesseracts! Nothing is more thrilling than seeing students experiencing math, and then turning their knowledge into something fabulous! I always reach for more ways to motivate students, and help them build their confidence in math...so they can keep learning and grow Math Esteem even further!

Resources

Here are a variety of helpful and supportive videos you may also want to check out:

https://youtu.be/Rob30oX9r4U

https://youtu.be/8fGWjBj0nD4

https://www.youtube.com/watch?v=iGO12Z5Lw8s